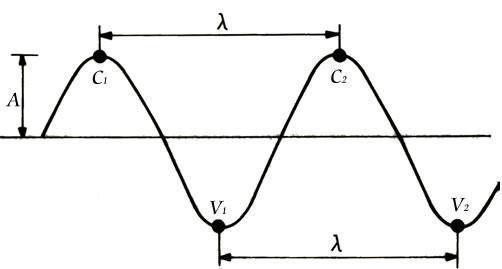
Uma onda transversal é aplicada sobre um fio preso pelas extremidades, usando-se um vibrador cuja frequência é de 85Hz. A distância média entre os pontos que praticamente não se movem é de 24cm. Então, a velocidade das ondas neste fio é de: A) 40,8 m/s B) 16 m/s C) 0,12 m/s D) 0,48 m/s E) 48 m/s
Respostas 1
A velocidade das ondas neste fio é de 40,8 m/s. Logo, a alternativa correta é a opção a) 40,8 m/s.
⠀
CálculoMatematicamente, a velocidade de uma onda é equivalente ao produto do comprimento da onda pela frequência, tal como a equação I abaixo:
[tex]\quad \LARGE {\boxed{\boxed{\begin{array}{lcr} \\\ {\sf v = \lambda \cdot f \ } ~\\\ \end{array}}}} \Large ~ ~ ~ \textsf{(equac{\!\!,}{\~a}o I)}[/tex]
[tex] \large \textsf{Onde:} [/tex]
[tex] \large \text{$\sf v \Rightarrow velocidade ~ da ~ onda ~ (em ~ m/s)$} [/tex]
[tex] \large \text{$\sf \lambda \Rightarrow comprimento ~ da ~ onda ~ (em ~ m)$} [/tex]
[tex] \large \text{$\sf f \Rightarrow frequ\hat{e}ncia ~ (em ~ Hz)$} [/tex]
⠀
AplicaçãoSabe-se, de acordo com o enunciado:
[tex]\LARGE \sf \displaystyle \rightarrow \begin{cases}\sf v = \textsf{? m/s} \\\sf \lambda = 2 \cdot 24~cm =\textsf{0,48 m} \\\sf f = \textsf{85 Hz} \\\end{cases}[/tex]
⠀
Assim, tem-se que:
[tex]\Large \text{$\sf v = \textsf{0,48} \left[m\right] \cdot 85 \left[Hz\right] $}[/tex]
[tex]\Large \text{$\sf v = \textsf{40,8} \left[m\right] \cdot \left[\dfrac{1}{s}\right] $}[/tex]
[tex]\boxed {\boxed {\Large \text{$\sf v = \textsf{40,8}\left[\dfrac{m}{s}\right] $}}}[/tex]
⠀
Leia mais sobre o assunto em:
brainly.com.br/tarefa/6572374
brainly.com.br/tarefa/13371757
brainly.com.br/tarefa/45791206
-
Autor:
chickie1dnj
-
Avalie uma resposta:
9