dada a f(x) =x2 -4x -12, faca oque se pedea) O vertice da funcaob)Os zeros da funcaoc) O esboco do grafico da funcao​

De acordo com os dados do enunciado e solucionado podemos afirmar que:

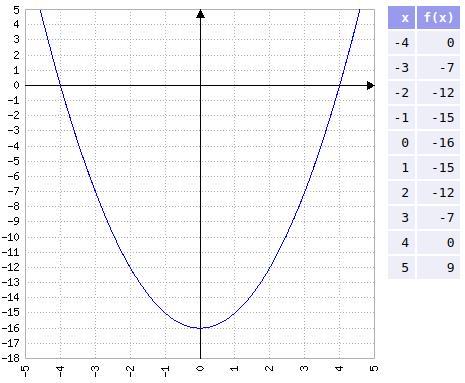
a) O vértice é o ponto V( 2, -16 );

b) Zeros da função: 6 e -2.

c) A figura em anexo.

A função quadrática (ou segundo grau), apresenta lei de formação y = f(x) = a.x² + bx + c, em a, b e c são os coeficientes da equação devendo ser números reais e a ≠ 0.

Zeros de uma função quadrática:

Os zeros ou raízes de uma função f(x) são os valores domínio para os quais f(x) = 0.

Vértice da parábola:

As coordenadas do vértice da parábola que representa a função do segundo f(x) =  ax² +bx +c é dada por:

[tex]\Large \displaystyle \text { $ \mathsf{ \begin{cases} \sf x_V = -\: \dfrac{b}{2a} \\ \\ \sf y_V = -\: \dfrac{\Delta }{4a} \end{cases} } $ }[/tex]

Dados fornecidos pelo enunciado:

[tex]\Large \displaystyle \text { $ \mathsf{ f(x) = x^{2} -4x -12 } $ }[/tex]

Resolução:

a) O vértice da função;

[tex]\Large \displaystyle \text { $ \mathsf{ x_V = -\: \dfrac{b}{2a} } $ }[/tex]

[tex]\Large \displaystyle \text { $ \mathsf{ x_V = -\: \dfrac{(-4)}{2\cdot 1} } $ }[/tex]

[tex]\Large \displaystyle \text { $ \mathsf{ x_V = \dfrac{4}{2} } $ }[/tex]

[tex]\Large \boldsymbol{ \displaystyle \sf x_V = 2 }[/tex]

[tex]\Large \displaystyle \text { $ \mathsf{ y_V = -\: \dfrac{\Delta }{4a} } $ }[/tex]

[tex]\Large \displaystyle \text { $ \mathsf{ y_V = -\: \dfrac{(b^{2} -4ac) }{4\cdot 1} } $ }[/tex]

[tex]\Large \displaystyle \text { $ \mathsf{ y_V = -\: \dfrac{((-4)^{2} -4\cdot 1 \cdot (-12)) }{4\cdot 1} } $ }[/tex]

[tex]\Large \displaystyle \text { $ \mathsf{ y_V = -\: \dfrac{(16 +48) }{4} } $ }[/tex]

[tex]\Large \displaystyle \text { $ \mathsf{ y_V = -\: \dfrac{64 }{4} } $ }[/tex]

[tex]\Large \boldsymbol{ \displaystyle \sf y_V = -\: 16 }[/tex]

b) Os zeros da função;

[tex]\Large \displaystyle \text { $ \mathsf{ x = \dfrac{-\,b \pm \sqrt{b^{2} -\, 4ac } }{2a} } $ }[/tex]

[tex]\Large \displaystyle \text { $ \mathsf{ x = \dfrac{-\:(-4) \pm \sqrt{(-4)^{2} -\, 4 \cdot 1 \cdot (-12) } }{2 \cdot 1} } $ }[/tex]

[tex]\Large \displaystyle \text { $ \mathsf{ x = \dfrac{4 \pm \sqrt{16 +48 } }{2 } } $ }[/tex]

[tex]\Large \displaystyle \text { $ \mathsf{ x = \dfrac{4 \pm \sqrt{64} }{2 } } $ }[/tex]

[tex]\Large \displaystyle \text { $ \mathsf{ x = \dfrac{4 \pm 8 }{2 } \Rightarrow\begin{cases} \sf x_1 = &\sf \dfrac{4+8}{2} = \dfrac{12}{2} = \: \: 6 \\\\ \sf x_2 = &\sf \dfrac{4-8}{2} = \dfrac{- 4}{2} = - 2\end{cases} } $ }[/tex]

c) O esboço do gráfico da função:

Em anexo.

Mais conhecimento acesse:

https://brainly.com.br/tarefa/49414412

https://brainly.com.br/tarefa/53470288